- Викладач: Geldhauser Nikita
- Викладач: Zhykhovich Maksim
- Викладач: Hainzl Christian
- Викладач: Madsen Peter
- Викладач: Panza Riccardo
- Викладач: Galli Leonardo
- Викладач: Nguegnang Gabin
- Викладач: Rauhut Holger
- Викладач: Gerkmann Ralf
- Викладач: Makai Tamas
- Викладач: Fono Adalbert
- Викладач: Gerkmann Ralf
- Викладач: Makai Tamas
- Викладач: Gritschacher Simon
- Викладач: Kotschick Dieter
- Викладач: Paul Laura
- Викладач: Terstiege Ulrich
- Викладач: Helling Robert
- Викладач: Phan Thành Nam
This is a continuation of the lecture course Functional Analysis from the previous semester. Attendance of the latter is not a necessary prerequisite for this course. However, a background in the theory of Banach and Hilbert spaces will be required. Main topics to be covered are a proof of the spectral theorem for bounded self-adjoint operators, an introduction to unbounded symmetric and self-adjoint operators and the basics of the Fourier transform and distributions.
Einschreibeschlüssel: selbstadjungiert
- Викладач: Müller Peter
- Викладач: Wetzel Leonard
- Викладач: Assy Lyn
- Викладач: Beyrer Jonas
- Викладач: Diesenberger Marie
- Викладач: Heißler Johannes
- Викладач: Kim Garam
- Викладач: Merkl Franz
- Викладач: Rast Arianna
- Викладач: Schoofs Benjamin
- Викладач: Araya Valdivia Ernesto
- Викладач: Seleznova Mariia
- Викладач: Bley Werner
- Викладач: Brenner Eva
- Викладач: Novikov Katharina
- Викладач: Willing Julius
- Викладач: Gritschacher Simon
- Викладач: Stelzig Jonas

- Викладач: Bowden Jonathan
- Викладач: Hensel Sebastian
- Викладач: Binder Karin
- Викладач: Hensel Sebastian
- Викладач: Söhnen Kajetan
Wir werden typische Aufgabenstellungen beim Staatsexamen in Analysis behandeln,
Lösungsmethoden besprechen und evtl. noch etwas zugrunde liegende Theorie
wiederholen. Wir beginnen mit dem Themenbereich
Gewöhnliche Differentialgleichungen und
arbeiten uns dann zur Funktionentheorie durch.
- Викладач: Zenk Heribert
Wir starten mit Anwendungen des Spektralsatzes wie
- stark kommutierende selbstadjungierte Operatoren
- Spektralsatz für stark kommutierende selbstadjungierte Operatoren
- stark stetige unitäre einparametrige Gruppen und deren Generatoren
- Викладач: Zenk Heribert
Mehrdimensionale Analysis -- Lehramt Gymnasium im Wintersemester 2024/2025
Dienstag 10-12 Uhr in B138 und Freitag 10-12 Uhr in B138
Beginn: Dienstag 15.10.2024
Die Vorlesung ist die dritte eines dreisemestrigen Kurses in Mathematik
für das Lehramt an Gymnasien nach der neuen Prüfungsordnung.
Einschreibung mit Schlüssel: ma
- Викладач: Darabi Far Fabian
- Викладач: Fiederer Marleen
- Викладач: Söhnen Kajetan
- Викладач: Zenk Heribert
Montag 12-14 Uhr in C123 und Donnerstag 10-12 Uhr
im Großen Physikhörsaal N120
Beginn: 14.10.2024
Die Vorlesung ist die erste eines dreisemestrigen Kurses in Mathematik
für das Physikstudium.
Am Montag 14.10. und 21.10. findet statt der Zentralübung
eine Vorlesung statt.
Einschreibung mit Schlüssel: MIP
- Викладач: Bauernfeind Matthias
- Викладач: Biber Veronika
- Викладач: Hallinger Cassian
- Викладач: Kugiyski Boyan
- Викладач: Papadopoulos Panagiotis
- Викладач: Zenk Heribert
The lecture provides an introduction to stochastic calculus with an emphasis on the mathematical concepts that are later used in the mathematical modeling of financial markets.
In the first part of the
lecture course the theory of stochastic integration with respect to
Brownian motion and Ito processes is developed. Important results such
as Girsanov's theorem and the martingale representation theorem are also
covered. The first part concludes with a chapter on the existence and
uniqueness of strong and weak solutions of stochastic differential
equations.
The second part of the lecture course gives an introduction to the arbitrage theory of financial markets in continuous time driven by Brownian motion. Key concepts are the absence of arbitrage, market completeness, and the risk neutral pricing and hedging of contingent claims. Particular attention will be given to the the Black-Scholes model and the famous Black-Scholes formula for pricing call and put options.
If you wish to participate in the course, please sign up by sending an e-mail from your LMU e-mail address to Annika Steibel (steibel@math.lmu.de).
- Викладач: Armayor Martínez Miguel
- Викладач: Karczewski Julia
- Викладач: Meyer-Brandis Thilo
- Викладач: Steibel Annika
- Викладач: Makai Tamas
- Викладач: Panagiotou Konstantinos
About this Lecture
This lecture discusses the interplay of Theory, Modelling, Numerical Methods and Implementation in Mathematical Finance.
All aspects learn from each other: one needs to understand the theory to build models and good implementations. Studying numerical experiments gives deeper theoretical insight. Using the computer to understand math can be fun!
We discuss how to build an industry-grade implementation of our models and allow future extensions while being efficient.
We discuss practical applications in the financial industry.
The lecture tries to be as self-contained as possible, but we will use some numerical methods developed in a previous course. We will start with a short recapitulation of the numerical methods needed for those who did not follow the last lecture. It is possible to consider most of these parts as “given” (“black box”). Don’t panic: We will assist you.
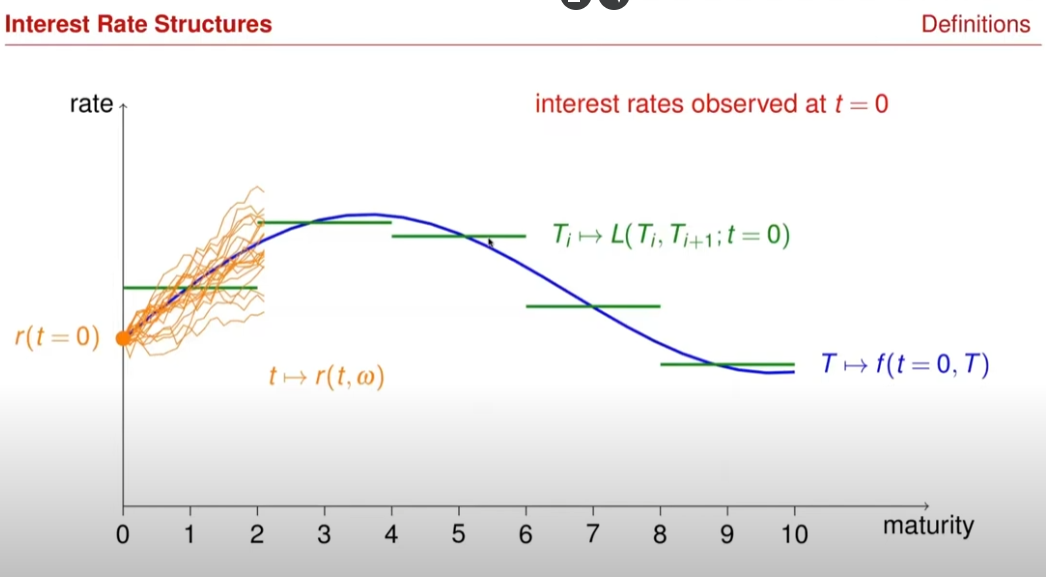
The lecture will discuss the theory and application of some prominent methods and models from mathematical finance. We focus on interest rate and hybrid models with high relevance for the financial industry. In an excursion, we consider a climate model (DICE), extend it and combine it with our interest rate models.
We will then use our implication to gain a deeper understanding of the theoretical properties of the model.
If time permits, we conclude the lecture by discussing running our models in a cloud.
Tentative Agenda
- Recapitulation of Numerical Methods (Monte-Carlo Method, SDEs, etc.)
- Interest Rates, Linear Interest Rates Products
- Multiple Interes Rate Curve Modelling and its Implementation
- Interest Rate Options, Convexity Adjustment
- Discrete Term-Structure Models (formerly known as LIBOR Market Models) and their Implementation
- Model Calibration
- Valuation of Complex Derivatives
- Object Oriented Implementation, Definition of Model Interfaces
- Short Rate Models and their Implementation
- Climate Models
- Numerical Methods in the Context of Mathematical Finance
- Model Calibration: Optimization and Root-Finding
- Correlation Modelling: Prinzipal Component Analysis and Factor Reduction, Regression Methods
- IT Implementation of Models, e.g. in the Cloud
The lecture covers the object oriented implementation of the algorithms and using modern software development tools.
As part of the implementation of the models and the valuation algorithms, the lecture will discuss some of the latest standards in software development.
- revision control systems (git)
- unit-testing (junit)
- build management (maven, gradle)
- continuous integration (TravisCI, Jenkins)
Implementation will be performed in Java (Eclipse, IntelliJ)
- Викладач: Fries Christian
- Викладач: Weber Niklas
Course Description
In this reading course, we will study stochastic processes in continuous time that happen to be sub- or supermartingales. In particular, this includes martingales. After proving Doob's maximal inequalities and stopping theorem, we will derive the quadratic variation of a local martingale. As a result, we will be able to understand the notion of a semimartingale.
Target Participants
- Master students of Mathematics
Pre-requisites
- Probability theory and measure and integration theory
- Викладач: Kalinin Alexander
Course Description
In this seminar, we will analyse one-dimensional stochastic differential equations (SDEs) driven by a Brownian motion. To this end, a short review of semimartingales, stochastic integrals and Itô's formula will be discussed. Under Lipschitz conditions on the drift and diffusion coefficients of the SDE, we will derive unique strong solutions. In particular, we will recover the Brownian bridge, the geometric Brownian motion and the Ornstein-Uhlenbeck process as solutions of affine SDEs.
Target Participants
- Master students of Mathematics and Financial and Insurance Mathematics
Pre-requisites
- Probability theory and measure and integration theory
- Викладач: Kalinin Alexander
Course Description
In this lecture, we will consider various classes of stochastic processes that may differ in their state spaces and underlying index sets with a special focus on Gaussian, Lévy and Markov processes. In summary, the lecture will be divided into three core topics: the construction, the path behaviour and the probabilistic analysis of general stochastic processes.
Target Participants
- Master students of Mathematics and Financial and Insurance Mathematics
Pre-requisites
- Probability theory and measure and integration theory
- Викладач: Kalinin Alexander
- Викладач: Bartolomaeus Wiebke
- Викладач: Reichert-Schürmer Paula
- Викладач: Reichert-Schürmer Paula
- Викладач: Ribelles Perez Anna
- Викладач: Sørensen Thomas
- Викладач: Fono Adalbert
- Викладач: Kutyniok Gitta
Einschreibeschlüssel: Newton24/25
- Викладач: Frank Rupert
- Викладач: Peteranderl Jonas
- Викладач: Pfeiffer Paul
- Викладач: Bollweg Karl-Wilhelm Georg
- Викладач: Oberpriller Katharina
Einschreibeschlüssel: Diff1WS24
- Викладач: Rost Daniel
- Викладач: Wenzel Tizian
Einschreibeschlüssel: LA1WS24
- Викладач: Böke Lukas
- Викладач: Paul Laura
- Викладач: Rost Daniel
Einschreibeschlüssel: Querschnitt24W
- Викладач: Schörner Erwin
- Викладач: Wetzel Leonard
Einschreibeschlüssel: Grundlagen1W24
- Викладач: Kolek Martinez De Azagra Stefan
- Викладач: Kolek Martinez de Azagra Stefan
- Викладач: Schörner Erwin
Einschreibeschlüssel: ExamUF24W
- Викладач: Rost Daniel
- Викладач: Schörner Erwin
Blockkurs in der vorlesungsfreien Zeit, in dem die Grundlagen des
Textsatzsystems LaTeX und speziell die Erstellung mathematischer Texte
behandelt werden. Nähere Informationen zu Ablauf und Anmeldung auf der Webseite des Kurses.
- Викладач: Callies Mechtild
Enrolment key: ICV
- Викладач: Giacomelli Emanuela
- Викладач: Land Markus
Inhalt der Vorlesung ist eine Einführung in die Optimierung in - vornehmlich - endlicher Dimension. Wichtige Themen und Inhalte sind unter anderem:
Konvexität, Lineare Programme und ihre Standardformen, Existenz von Lösungen für lineare Programme, Dualitätstheorie für lineare Programme, das Simplexverfahren, Formulierung und Existenz von Lösungen konvexer Optimierungsprobleme, duale Darstellung konvexer Funktionen und die Kuhn-Tucker-Theorie.
- Викладач: Becker Julian
- Викладач: Panagiotou Konstantinos
Wir beginnen mit einer Einführung in die klassische Algebraische Geometrie der affinen und projektiven Varietäten und deren Morphismen. Zu den weiteren Themen gehören rationale Abbildungen, Dimension, Vollständigkeit, Tangentialräume, singuläre Punkte, Normalisierung und Aufblasungen.
Im Anschluss geben wir eine Einführung in die Theorie der Garben und Schemata.
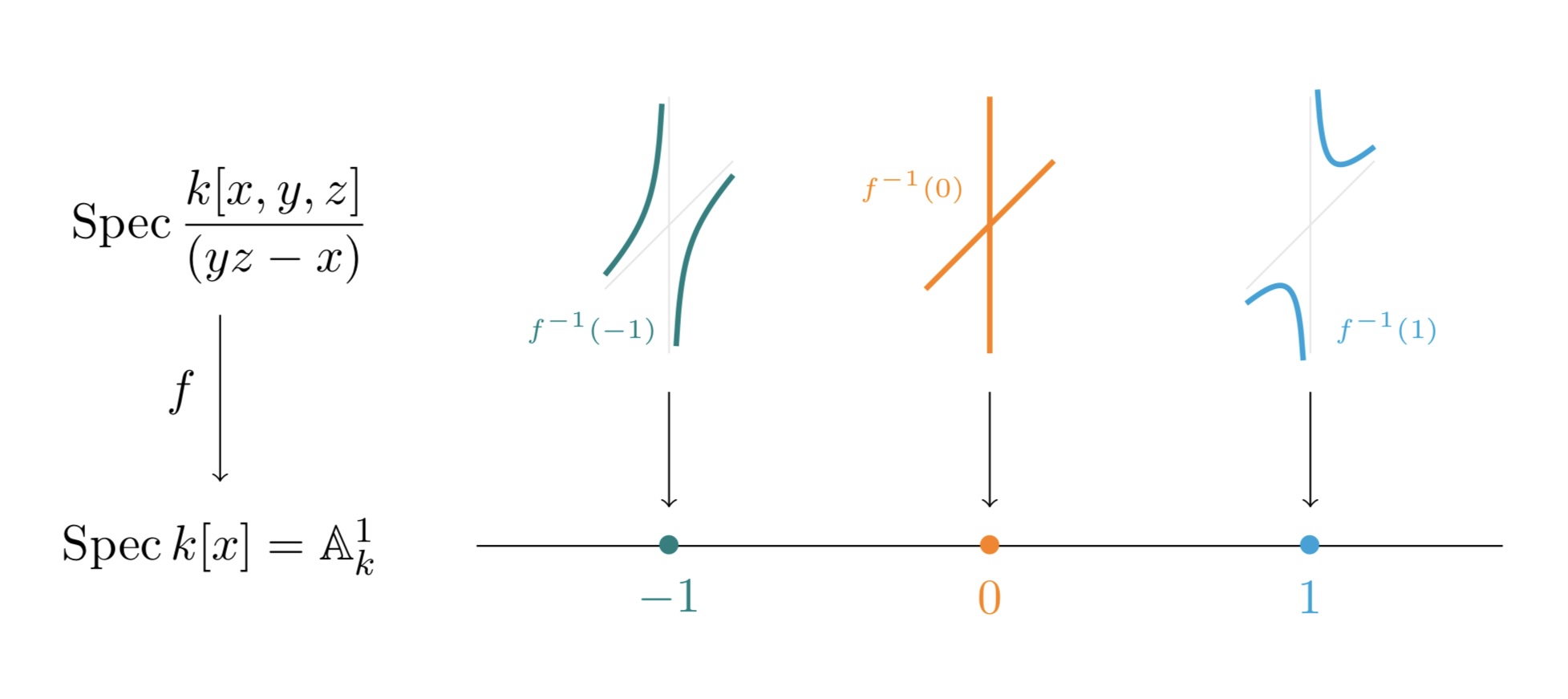
- Викладач: Rosenschon Andreas
- Викладач: Seidenschwarz Simon
- Викладач: Beyrer Jonas
- Викладач: Lange Christian
- Викладач: Leeb Bernhard
Passwort: cantelli
- Викладач: Jansen Sabine
- Викладач: Nolte Fabian

- Викладач: Bartenschlager Johannes
- Викладач: Bowden Jonathan
Einschreibung öffnet am 7. Oktober 2024.
Klausuranmeldung (in 2025) ausschließlich über Moodle.
- Викладач: Bollmann Leon
- Викладач: Duell Maximilian
- Викладач: Sørensen Thomas